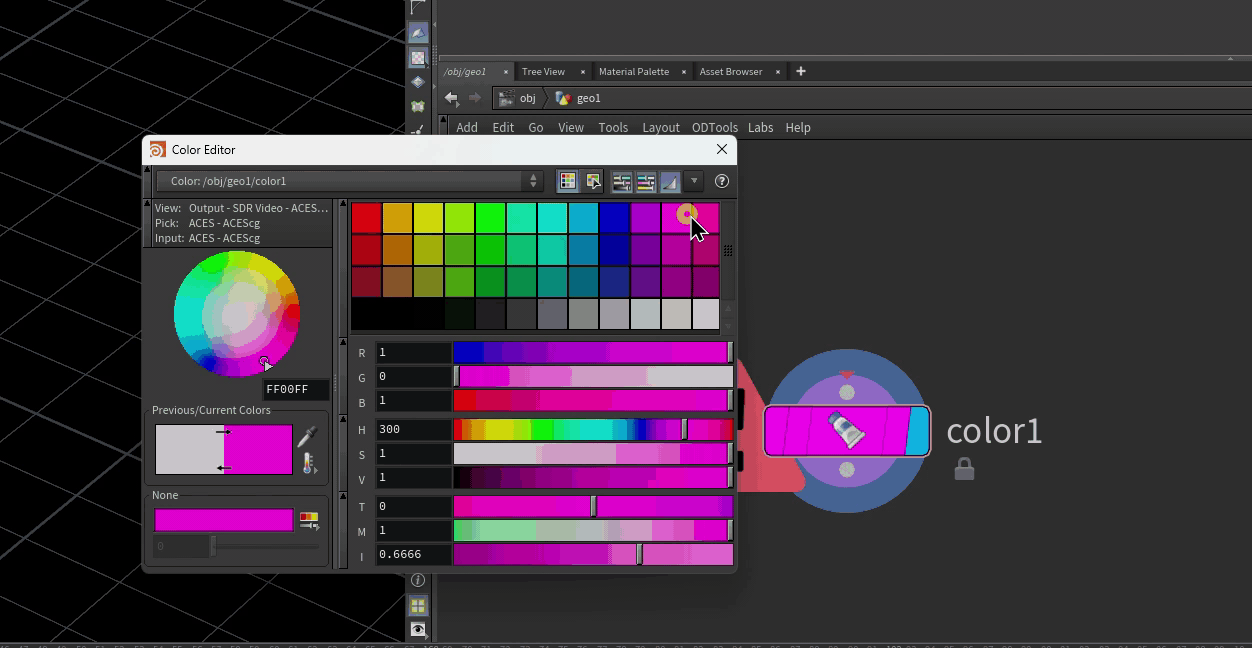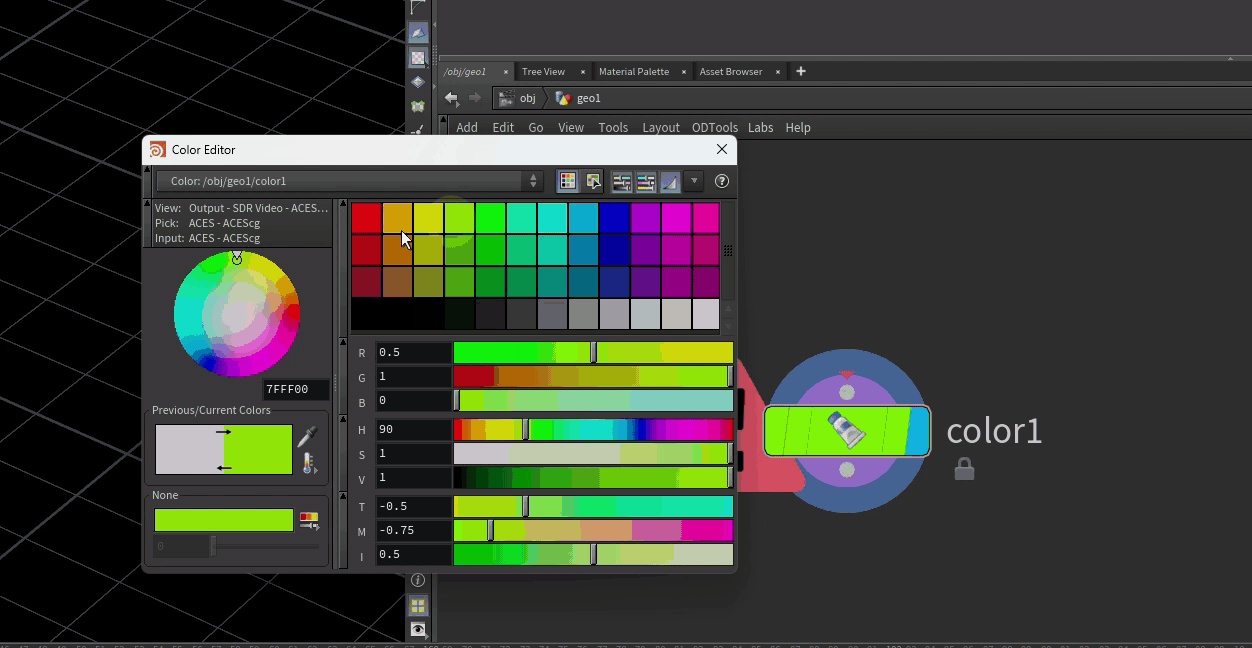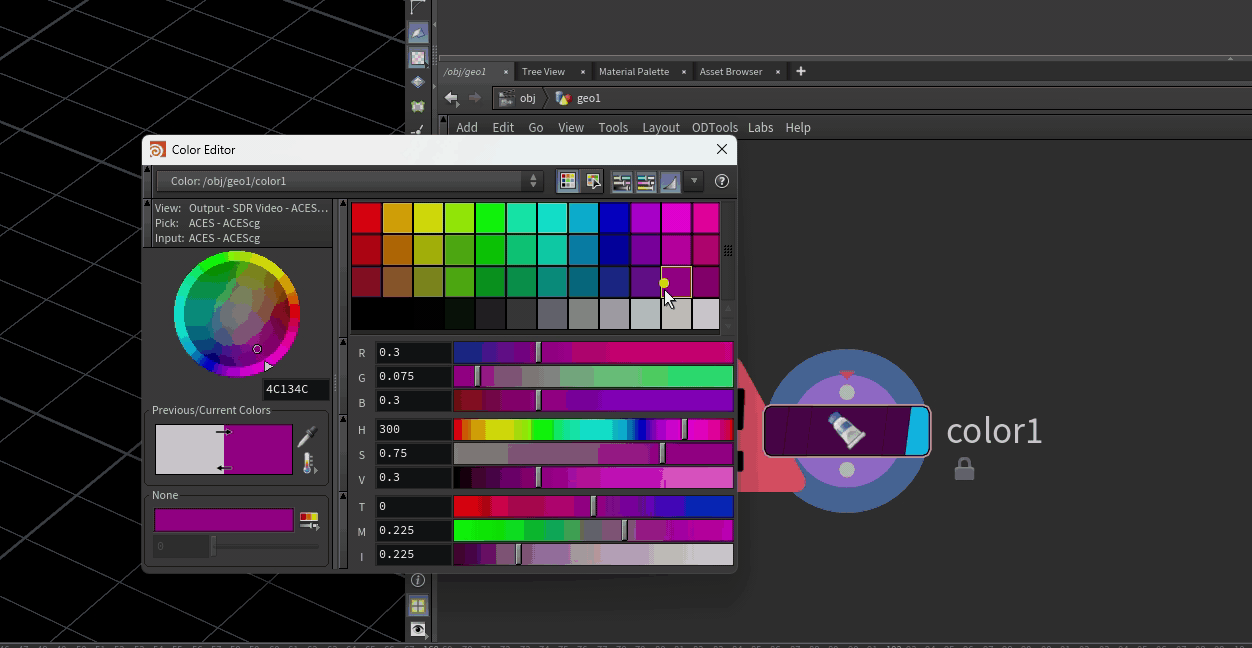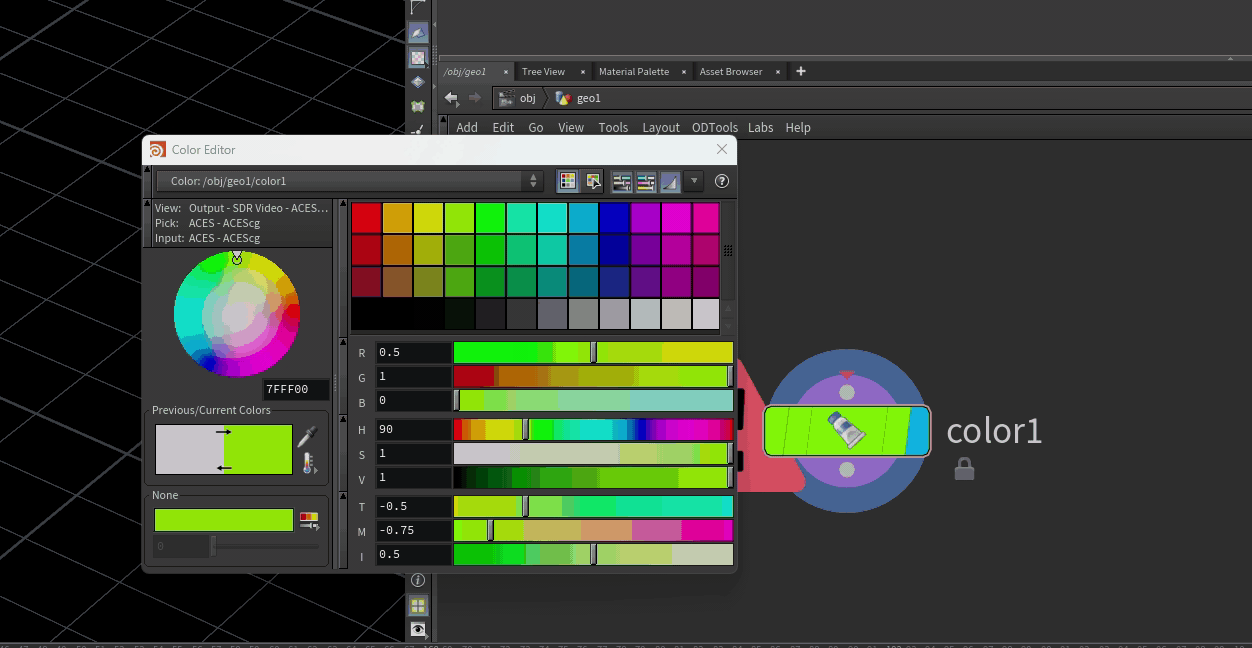
Casey Hupke asked an interesting question today: “how can I get a Color SOP to automatically update its node color to match the picked color?” This reminded me of some other pipeline work I’d done in the past to customize existing nodes in Houdini; for example, adding a few spare Read more…
The big draw of The Conservatory, other than the very neat rain shader, is the plants. I spent a long time researching different candidate plants that would roughly fit into the same tropical environment, with a decent range of morphologies that would help fill out the space. That meant a Read more…
Those of us unlucky enough to be rendering stills for print are familiar with the idea of rendering tiles in order to allow a render job to be more easily parallelized. Instead of trying to render and save one massive 20k square image to disk from a single machine, bucket Read more…
In case you haven’t seen it already, my presentation on MOPs at Houdini Hive Worldwide has been online for about a month now. Here’s the presentation: I’d forgotten to upload the example files I built during the presentation. They’ll be included in the examples directory in the next Experimental release Read more…
This is a sort of sequel to the previous post I made about handling the Houdini.env file, and about configuring environments in general. Houdini 17.5 introduced the concept of “packages”, which are little JSON files (JSON meaning JavaScript Object Notation, filling a similar role as XML or YAML) that define Read more…
Another year, another very very short side project. This was meant to be both an exploration of Vellum (and an attempt to see if I could force Vellum to work in 2D), and a neat little intro video for a new demo reel. This project is considerably less complicated than Read more…
SideFX Software was generous enough to ship me out to the Netherlands last week to meet my partner-in-MOPs, the inimitable Moritz Schwind, and present together a little slide show about motion graphics, Houdini, and prison hooch at End User Event 2019! The technical stuff about MOPs starts about halfway through Read more…
Update: Imre Tuske from Weta pointed out that there’s a simpler method… it turns out that you can force expressions to evaluate inside a Wrangle by wrapping them up in backticks. So if you have a channel called “do_vexpressions” to enable or disable VEXpressions, and another channel called “vexpression” that Read more…
After months of work, the MOPs toolkit for Houdini is finally open to the public! MOPs is a suite of SOP tools to help with common motion graphics tasks in Houdini. The workflow and interface should seem familiar to users of other motion graphics software packages, but it’s using Houdini-friendly Read more…
In case you missed it, part 1 is here (and includes the .hip file!). Part 2 is here. In this last part of the breakdown, I’m going to talk about some of the smaller little details of the piece: the background plants, called “sprouts” in the file, the dewdrops, and Read more…